 /
/ 
実行時間制限: 2 sec / メモリ制限: 256 MiB
配点 : 700 点
問題文
すぬけ君は、パズルゲームで遊んでいます。 このパズルゲームでは、R × C の長方形の盤面に、1 から N までの整数が 2 つずつ書かれています。 整数 i が書かれている座標は、(x_{i,1},y_{i,1}) と (x_{i,2},y_{i,2}) です。
すぬけ君の目的は、1 から N までのすべての整数に対し、同じ整数の書かれている座標同士を曲線で結ぶことです。 このとき、曲線が長方形の外に出たり、互いに交わったりしてはいけません。
このようなことが可能かどうか判定してください。
制約
- 1 ≦ R,C ≦ 10^8
- 1 ≦ N ≦ 10^5
- 0 ≦ x_{i,1},x_{i,2} ≦ R(1 ≦ i ≦ N)
- 0 ≦ y_{i,1},y_{i,2} ≦ C(1 ≦ i ≦ N)
- 与えられるどの 2 点も異なる
- 入力は全て整数である
入力
入力は以下の形式で標準入力から与えられる。
R C N
x_{1,1} y_{1,1} x_{1,2} y_{1,2}
:
x_{N,1} y_{N,1} x_{N,2} y_{N,2}
出力
すぬけ君が目的を達成できるなら YES を、そうでないなら NO を出力せよ。
入力例 1
4 2 3 0 1 3 1 1 1 4 1 2 0 2 2
出力例 1
YES
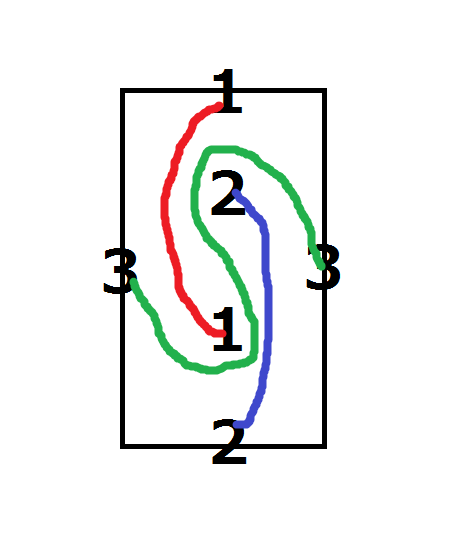
上図のように整数同士を結べば、目的を達成することができます。
入力例 2
2 2 4 0 0 2 2 2 0 0 1 0 2 1 2 1 1 2 1
出力例 2
NO
入力例 3
5 5 7 0 0 2 4 2 3 4 5 3 5 5 2 5 5 5 4 0 3 5 1 2 2 4 4 0 5 4 1
出力例 3
YES
入力例 4
1 1 2 0 0 1 1 1 0 0 1
出力例 4
NO
Score : 700 points
Problem Statement
Snuke is playing a puzzle game. In this game, you are given a rectangular board of dimensions R × C, filled with numbers. Each integer i from 1 through N is written twice, at the coordinates (x_{i,1},y_{i,1}) and (x_{i,2},y_{i,2}).
The objective is to draw a curve connecting the pair of points where the same integer is written, for every integer from 1 through N. Here, the curves may not go outside the board or cross each other.
Determine whether this is possible.
Constraints
- 1 ≤ R,C ≤ 10^8
- 1 ≤ N ≤ 10^5
- 0 ≤ x_{i,1},x_{i,2} ≤ R(1 ≤ i ≤ N)
- 0 ≤ y_{i,1},y_{i,2} ≤ C(1 ≤ i ≤ N)
- All given points are distinct.
- All input values are integers.
Input
Input is given from Standard Input in the following format:
R C N
x_{1,1} y_{1,1} x_{1,2} y_{1,2}
:
x_{N,1} y_{N,1} x_{N,2} y_{N,2}
Output
Print YES if the objective is achievable; print NO otherwise.
Sample Input 1
4 2 3 0 1 3 1 1 1 4 1 2 0 2 2
Sample Output 1
YES

The above figure shows a possible solution.
Sample Input 2
2 2 4 0 0 2 2 2 0 0 1 0 2 1 2 1 1 2 1
Sample Output 2
NO
Sample Input 3
5 5 7 0 0 2 4 2 3 4 5 3 5 5 2 5 5 5 4 0 3 5 1 2 2 4 4 0 5 4 1
Sample Output 3
YES
Sample Input 4
1 1 2 0 0 1 1 1 0 0 1
Sample Output 4
NO